


Next: The Palm Distributions P P!
Up: Stationary Marked Point Processes
Previous: The Palm Mark Distribution
Consider a so-called averaging sequence 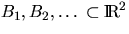 of test sets such
that
Bn is convex and compact,
of test sets such
that
Bn is convex and compact,
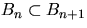 for each
for each 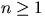 , and
, and
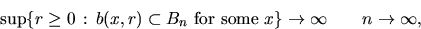
where b(x,r) denotes the circle with radius r and
center at x. Then in many cases of practical interest we have with
probability 1
| 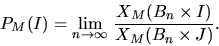 |
(3) |
We remark that a sufficient condition for (3.3) is that
(X,M) is ergodic.
In particular, (3.3) holds if (X,M) is an independently marked
Poisson process. Other examples where (3.3) is true are marked
point processes which are induced by a homogeneous Poisson process
(or an arbitrary stationary ergodic point process) via a
shift-compatible mapping of its realizations; see Daley and Vere-Jones (1988). Such marked point processes
will be considered e.g. in Section 5, in connection with
random tessellations of the plane.
Equation (3.3) gives the motivation to say that PM is the
mark distribution of the typical point of (X,M). For keeping
the terminology simple, we use this way of speaking also in the case
when we do not assume that the limit in (3.3) is constant
with probability 1. Note, however, that this interpretation of the Palm
mark distribution is then not fully justified.
In the case when the marks are nonnegative random variables,
the expectation of PM will be denoted by 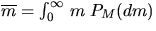 and called the expected
mark of the typical point.
and called the expected
mark of the typical point.



Next: The Palm Distributions P P!
Up: Stationary Marked Point Processes
Previous: The Palm Mark Distribution
Andreas Frey
7/8/1998
![]()
![]() and called the expected
mark of the typical point.
and called the expected
mark of the typical point.